Numerical sets and complex numbers

Numerical sets

The numbers obtained by counting objects are called natural numbers. The numerical set of natural numbers is designated by the letter N, N = {1,2,3,...}. If we add the number 0 and negative integers to the set N, we get the set of integers. It is denoted by Z, Z =  The set of rational numbers is denoted by the letter Q, Q = {m/n, m∈Z, n∈N}. A rational number can always be represented as a finite decimal fraction or infinite periodic decimal fraction. In addition to rational numbers, there are numbers that can be represented as an infinite non-periodic decimal fraction, for example, √2 = 1.41421..., π = 3.14159... . Such numbers are called irrational. If we add the set of irrational numbers to the set of rational numbers, we get the set of real numbers. It is denoted by the letter R. The sets N, Z, Q are subsets of the set of real numbers R.

Complex numbers

Not every quadratic equation has solutions in the real number domain, for example, x² + 1 = 0. It is possible to expand the real number domain so that every quadratic equation has a solution. Such a set is the set of complex numbers. It is denoted by the letter C, and complex numbers are expressions of the form z = a + bi, where a and b real numbers, i = √-1 - imaginary unit for which the equality holds i² = √-1. The number a is called the real part of the number z (a=Re(Z)), and b is called the imaginary part (b=Im(z)). If b=0, then z is a real number. If a=0, then z is called a purely imaginary number. For the imaginary unit, the following equalities are valid:

i²= -1;   i³=i²i=-i;   i⁴=i²i²=1;   i⁵=i⁴i=i; ...

The concepts of "more", "less" do not exist for complex numbers. But we can talk about the equality or inequality of two complex numbers. Two complex numbers are equal to each other if and only if their real and imaginary parts are equal.

Algebraic notation of complex numbers

The notation of the form z = a + bi is called the algebraic form of a complex number. The opposite number for the number z = a + bi is the number z = -a - bi.
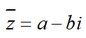The complex conjugate of the number z = a + bi is the number The number line is used to represent all real numbers. Complex numbers z = a + bi are represented by points on a plane with coordinates (a,b) in the chosen coordinate system. This plane is called complex. The X axis is the axis of real numbers, on it are the numbers (a;0). The Y axis is the axis of imaginary numbers, on it are the numbers (0;b). Any point (a,b) in this coordinate system corresponds to a complex number z = a + bi and, conversely, to each complex number z = a + bi corresponds to point (a;b) on the complex plane.
Thus, a complex number can also be viewed as an ordered pair of real numbers (a;b)=a+bi.